Modeling Thermocouples with FLOTHERM

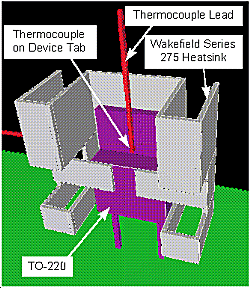
Did you ever wonder what effect a thermocouple has on your experimental data? Can you trust the measured temperature or is there a hidden source of error you have not considered? Dr. Cathy Biber, Senior Thermal Engineer with Wakefield Engineering and FLOTHERM user, recently addressed this topic. She began by building a model of a Wakefield series 275 heat sink attached to a TO-220 power semiconductor dissipating 3 watts in a natural convection environment. Two thermocouples were added to the model, one on the heat sink and one on the device tab as shown below.

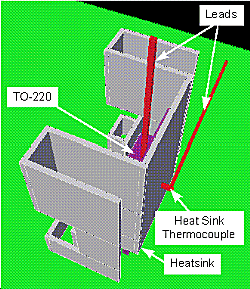
Dr. Biber's method for modeling a thermocouple (TC) consists of three parts: the bead, the wire and the contact resistance between the bead and the surface whose temperature is being measured. The bead and wire should be modeled as solve-in-solid cuboid blocks, with appropriate thermal conductivity to account for the type of TC being used in the experiment. Remember to calculate an equivalent thermal conductivity for the bimetallic wire. As an example, Dr. Biber used a T type (copper-constantan) thermocouple which worked out to have an equivalent thermal conductivity of 100 W/mK.
 

Finally, an internal plate is used to model the contact resistance between the bead and the surface. The thickness and thermal conductivity of the plate are critical in obtaining agreement with test results. Cathy Biber used the conductivity of air (0.026 W/mK) and a thickness of 1.5 microns to represent the contact resistance in her model. However, it is important to model the effects of thermal grease or adhesives if used. The routing of the TC wire in your model should match that of the experimental set-up.
 

Results

Experimental results were compared to FLOTHERM model predictions with and without the presence of thermocouples. In the model without thermocouples, the temperature was taken from a cell within the device tab and within the heat sink. In the model with thermocouples, the temperature was taken from the cells representing the TC beads. The following table presents the results.
 

As you can see, the model containing thermocouples has excellent agreement with the experimental data. This model was correlated to one set of experimental results and then tested against several other sets of data, all with similar agreement. However, in the model without TC's, the results showed temperature differences of 6C on the device and 13C on the heat sink!

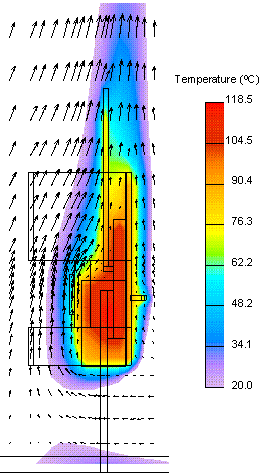
Conclusions

Two points are clear as a result of this exercise:

• "Just because you've measured it, that doesn't make it right". There are errors and inaccuracies involved with testing as well as with modeling.
• If you are having difficulty correlating your model with test results, don't dismiss your model as inherently inaccurate. It may simply be that you have not accurately captured the particulars of the test setup.