Estimating the Influence of PCB and Component Thermal Conductivity on Component Temperatures in Natural Convection

What is a good value of thermal conductivity to use in FLOTHERM simulations for printed circuit boards (PCBs) and components if you have no real data?

10 watt/m/C.

A method of predicting component temperatures.

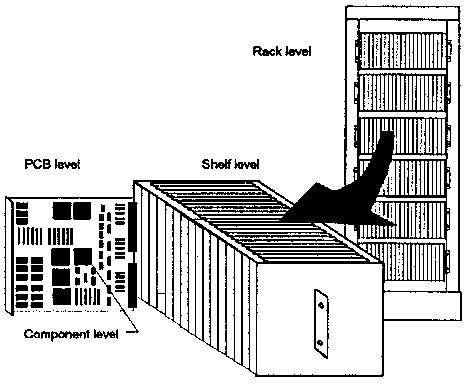
The typical thermal simulation problem that comes up at Tellabs is shown in Figure 1. There are from one to a few PCBs mounted vertically in a shelf, cooled by natural convection, with air entering through vents in the bottom and exiting through similar vents in the top. On each PCB there are only a few thermally important components, in the sense that they dissipate an appreciable amount of heat, and several hundred components that each dissipate a trivial amount of power, but together, may dissipate a few wafts. I am interested in the temperatures of only the important components, knowing that if they are OK, the trivial components will only be cooler It would be nice when constructing the FLOTHERM model of each PCB, to be able to treat the trivial components as uniform lumps of heat dissipating stuff, and to model in detail only the components that must be known most accurately.

By trial and error I have developed a method for modeling PCBs in natural convection. The first step is to divide the components into two sets: Important and Trivial. Important components are those for which I want to know the temperature. Either they are high power, or temperature sensitive. Trivial components are all the rest. They are low power and are not worth modeling in detail, because I already know they will not be hot. But the power they add to the board, and the flow resistance they cause will not be ignored. Almost everything is a Solve-in-Solid Cuboid Block. The PCB, the important components and the trivial components are all Solve-in-Solid Cuboid Blocks. Internal Plates and FOB elements are only rarely used, because in natural convection problems, temperature is not very uniform, and conduction in all three directions can be very important. Internal Plates do not conduct heat along their surfaces, and POB elements do not conduct heat perpendicular to their surfaces.

Models of Trivial components must have the following characteristics: they block air flow; they contribute heat by conduction into the board; they act as heat dissipating surfaces to the air, possibly conducting heat between the board and the air; and they contribute heat to the air stream, causing some portion of the air flow. All these things they should do in a reasonable approximation of the individual components. By reasonable I mean not totally accurately, because I am not concerned with predicting the temperatures of these parts. I am concerned that the effect of Trivial components on the temperature of the Important components is well modeled.

The easiest way to do this is to identify patches of the POE populated with Trivial components that are all about the same height, to draw a cube around them, to add up all the individual power dissipations, and to assign that power to the Solve-in-Solid Cuboid Block. The accuracy 'with which the Block must represent the individual components is more important near the Important components, and not very important far away.

Each Important component is also modeled as a Solve-in-Solid Cuboid Block. I draw a cube that just encloses the component, and is flush to the PCB, unless the real component is mounted high off the PCB. The normal tiny gap under DIPs and surface mount parts is usually neglected. I do not try to model details such as leads, lead frames or the die inside the package, because the properties and geometry are generally not known, and would require too much detail (and a very fine grid) if a whole PCB were modeled this way. The power dissipation is assigned to the whole block uniformly.

The PCB is a Solve-in-Solid Cuboid Block with no power dissipation. Unlike an Internal Plate, it allows heat conduction from one component to its neighbors. Unlike the simple FLOTHERM PCB element, the Solve-in-Solid Cuboid Block POE conducts heat from one side of the board to the other. My experience is that the simplified representation of boards by Internal Plates and PCB elements is just not adequate for natural convection. It tends to overpredict component and air temperatures.

There is one characteristic of the Solve-in-Solid Cuboid Blocks that I have not talked about yet which is very important in getting this modeling technique to work. That is choosing the thermal conductivity, which is the original question above.

Each component and the PCB itself is a three-dimensional composite of different materials with vastly different thermal conductivity. The conductivity of copper is three orders of magnitude greater than that of epoxy resin or the plastic package compound. It is not a simple thing to look at a 28 pin DIP and come up with a single lumped together value of thermal conductivity. It is also not obvious how to calculate the lumped conductivity of a PCB that may not even have been designed yet. If one has a finished PCB design, it is at least possible to estimate the ratio of copper to epoxy.

Analytical approach to conductivity.

Every undergrad heat transfer text shows how to calculate the effective conductivity of a composite solid. For the simplest case of a PCB made up of alternating layers of epoxy and copper, the thermal conductivity in the plane of the PCB is given by:

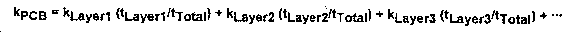where k_n is the conductivity of the nth layer and t_n is the thickness of the nth layer.

For a double-sided PCB with solid layers of 1 ounce copper on the top and bottom with a total board thickness of 1.59mm we can estimate a board conductivity of:

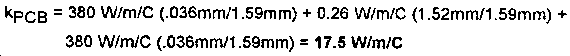The conductivity of the PCB perpendicular to the plane of the board in this example is not affected much by the presence of the copper layers, and is still only about 0.26 W/m/C. A real board has numerous vias that conduct perpendicular to the board, and if the via density is known, an effective conductivity in the perpendicular direction can be calculated. A real board does not have totally solid signal and ground layers, and the effect of signal layers may have at least some local effect on board conductivity. The simple calculation above gives us a starting point, at least in order of magnitude, for searching for values of k_PCB and kcomponent that allow this modeling method to predict component temperatures accurately.

HDSL.

The first evidence that this method might work turned up on a project called HDSL. Figure 2 shows why the design team was anxious to simulate this product before freezing the design. It is a three-board sandwich, surrounded by a metal shield. The holes in the shield were minimized to prevent electromagnetic interference (EMI) problems. It is cooled by natural convection, and can be mounted in an environment up to 650. We not only wanted to predict component temperatures, but to know the effect of vent hole size on temperature, and how many shelves of these modules could be safely stacked. 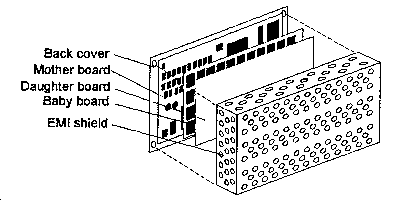The design engineers had calculated realistic power dissipation for the components in the module, which meant that if any simulation had a chance of predicting accurate temperatures, this one did.

We were particularly interested in two components, code-named Processor and Timber. Processor and Timber were modeled individually, and all the other components were lumped together in large cuboid blocks. Thermal conductivity for all the components and the PCBs was set at 10 W/m/C. After running the FLOTHERM simulation, refining the grid, and getting the best possible temperature results, I showed the model to the team rather cautiously, because it predicted that Processor would be too hot. Not willing to accept bad news, the team sharpened its collective pencil, recalculated the power estimates, and cut them nearly in half. That knocked more than 20C off Processor's temperature, which made us happy, and several months later, when a prototype was tested, I was pleasantly shocked at the results in Table 1.

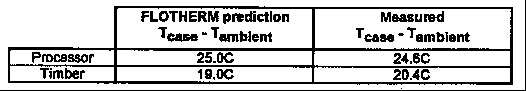
These results appear almost too good to be true, and because only two components were modeled in detail, it was impossible to tell if the match of prediction with measurement was only a lucky coincidence. This early result did teach me that it would not be possible to determine a useful value of thermal conductivity for PCBs and components unless component power dissipation is known accurately. In the HDSL model, the thermal conductivity was chosen because it was in the realistic range between 1 and 100 W/m/C hinted at by the analytical approach. After the experimental results came so close to the predicted values, 10 became a number to keep in mind.

Power supply board Figure 3 shows an extreme application of the method of combining components together into large Solve-in-Solid Cuboid Blocks. This board is a DC-to-DC power converter that supplies the rest of the PCBs in the shelf from -48V input. In the FLOTHERM model I lumped together all the components, except for one relay, into a single Solve-in-Solid Cuboid Block that covers almost the entire board. The relay is modeled as another Solve-in-Solid Cuboid Block. This model, of course, is not very useful in predicting component temperatures on the power supply board, but in this case, that was not my purpose. The power supply had already been built, and I already knew all the component temperatures by measurement. What I did not have were multiple copies of the power supply, and the problem that I needed to simulate was how many shelves could be stacked vertically and still be cooled safely by natural convection. The lame Solve-in-Solid Cuboid Block would add the heat to the air stream, causing air temperature to rise from one shelf to the next. The relay block represented a "typical" component on the board, to give me some idea how component temperatures would rise from shelf to shelf: I chose the relay because it was one of the hottest parts on the board, and its power dissipation was fairly well known. Although the total dissipation of the rest of the board was known accurately by measuring the input and output currents, the dissipation of the individual analog components was almost impossible to determine.

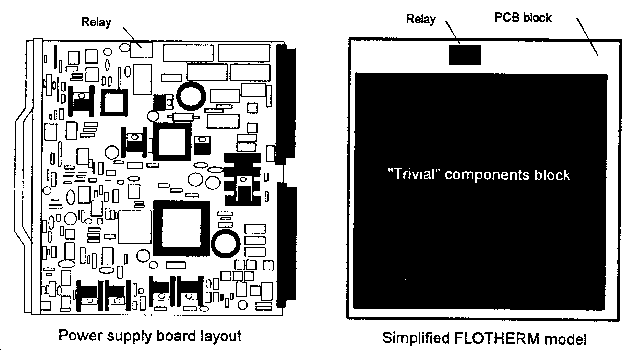
Again the value of 10 W/m/C was used for the PCB as well as the two Cuboid Blocks. For the single shelf case (which was the only case that could be verified experimentally), the temperature results were:

This is not a landslide of data. It was not intended to be at the time. However, this one data point gave me the feeling that there were at least a few things right in the model: the power dissipation, the physical geometry, and the thermal conductivity of the solids. This gave confidence that growing the model by stacking additional shelves would have a good chance of being accurate, or at least a much better chance than a model of a system of shelves that had no grounding at all in experimental data.

At this point I might even accuse myself of picking and choosing my case studies to show only the most favorable results. It is true that I have been picking and choosing. My FLOTHERM casebook is full of temperature predictions, and my lab notebook is full of temperature measurements, and it is very difficult to find numbers that match. The general trend is that the predictions are somewhat higher than the measurements. I believe that the most important reason for this is the overestimation of power dissipation for components in the models. The criterion I used for selecting the cases from my records was whether component power dissipation was well defined. The simple "I_cc (max)" from the manufacturers' data sheets was not good enough.

Typhoon.

There was a real shortage of cases in which component power dissipation was well defined. For the purpose of this study I worked a simulation problem backwards. I found a board (project name Typhoon), which had not be simulated, but for which I had measured the temperatures of the important components. This board was chosen because the power had been determined fairly accurately for at least the Important components. I estimated the power for all the rest of the components as realistically as possible, avoiding the use of "max power" figures. Then I built a FLOTHERM model of the Typhoon board, using the technique described before. Figure 4 shows how Trivial components were lumped together in the model.

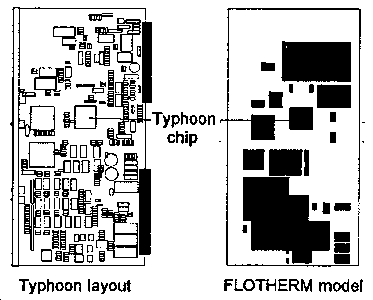
All the conductivities were originally assigned as 10 W/m/C, and the grid was refined until the component temperature change was less than 10. Then various combinations of PCB and component thermal conductivity were tried to see which would make the best match between component temperature predictions and the temperature measurements. Only the Important components were considered in the correlation, because it was assumed that the temperature of the large, lumped-together blocks would not accurately represent any one component in them. The comparisons are shown in Table 3.

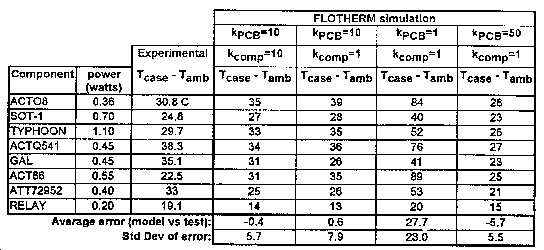
This PCB had lots of copper: four signal layers, a power plane and a ground plane. It makes sense that the PCB conductivity should be closer to 10 W/m/C than to 1 or to 50, as borne out by Table 3. Table 3 also seems to indicate that the conductivity of the individual components is not as important as the PCB conductivity, as long as it is in the range between 1 and 10 W/m/C. That is probably because power is dissipated uniformly within the volume of the Cuboid Blocks, leading to little or no temperature gradient inside the blocks.

This study seemed more realistic than the first two examples given above, because no single component matched prediction and measurement exactly under any condition. Also, adjusting the single parameter of conductivity had the expected effect on the average error for all the important components, rather than just for one or two components.

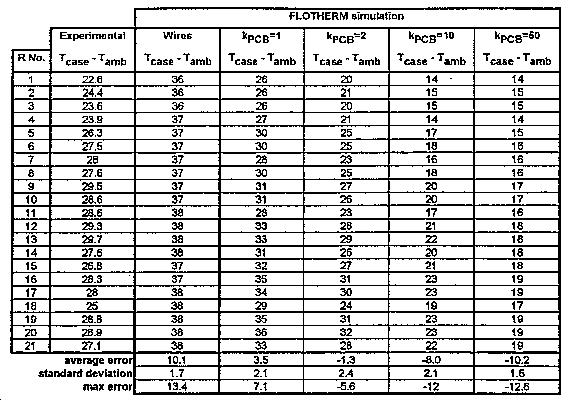
Resistor Board.

Even in the Typhoon board case, the component power was only estimated, not measured. Figure 5 shows a board that did not have this problem, a bare epoxy board with an array of wirewound power resistors. The resistors were connected in series with discrete, uninsulated wires, not printed wire. The actual power dissipation of each resistor could be measured while the temperature measurements were being made. It would also be possible to model in detail the wires and the board without any approximated thermal conductivity, because the geometry was very simple and the material properties well known.

In the experiment, a total of five watts was applied to the resistor board. Five watts for a board of this size is typical for products cooled by natural convection. Case temperature of each resistor was measured by thermocouple. The power of each resistor was confirmed by measuring the voltage drop across each after they had reached steady state temperature under power.

Two types of FLOTHERM models were built. The first attempted to simulate physical reality as closely as possible. The board was a Solve-in-Solid Cuboid Block with k = 0.2 W/m/C. Each of the 21 resistors was a Solve-in-Solid Cuboid Block with k = 10 W/m/C with a uniform power dissipation of 0.238 watts. The wire connecting them in series was also modeled as a Solve-in-Solid Cuboid Block with k = 200 VV/m/C. The only large departure from reality was approximating the cylindrical shapes of the resistor bodies and the wires as cuboids. The dimensions of the cuboids were adjusted to give the same surface area as the original cylinders.

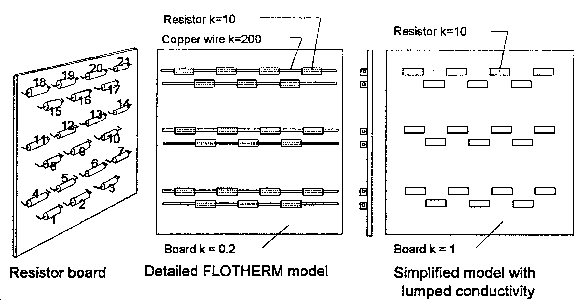
The second type of FLOTHERM model was a simplification of the board and wires, lumping them together and assigning a composite thermal conductivity to the board. This is the method used in the first three cases above, where modeling the details of the printed wiring was impractical. In this case it was expected that the simplified model would have more error that the more detailed, more realistic model. The thermal conductivity of the real board was very non-uniform: the wire connecting the resistors snakes across the board in a way that should create small areas of high conductivity and large patches of very low conductivity. The results in Table 4 show some surprises.

The column marked "Wires" is the detailed model that modeled the wires and the board separately. It tended to overpredict the resistor temperatures by about 10C.

The surprise here is that the simplified model with the composite value of thermal conductivity actually does a better job of matching the experimental results. The best value of k for the board is about 1 or 2 W/m/C. It is expected that a value lower than the magic 10 W/m/C should work better in this case, because the amount of copper involved is quite a bit less, and it is not uniformly distributed around and through the board.

Board conductivity higher than 2 W/m/C leads FLOTHERM to predict lower and lower resistor temperatures. The negative sign for the average error in these simulations indicates that the prediction is lower than the measurement. Setting the value of board conductivity "too high" in this simulation causes too much heat to spread into the board from the resistors, leading to unrealistically low component temperatures. This type of error is probably more dangerous than overpredicting component temperatures.

The conclusions of this paper are more in the nature of reporting my experience with PCB thermal conductivity, rather than a rigorous scientific experiment. I have carefully chosen the cases that were reported, 'with the best of intentions, but the very fact that I chose to report some cases and not others means that my personal bias cannot be excluded. Assuming that the work I have done is valid, there are still some limitations to its application:

• All of the cases studied are in natural convection. I don't know whether this method of lumping thermal conductivity, or the generally useful value of 10 W/m/C, would work well under forced cooling conditions. It is my guess that conduction in the PCB becomes less important as air velocity and heat transfer to the air stream go up, and so the importance of having an accurate value of conductivity is not as important.



• The average power dissipation was less than 100 W/m² (counting the surface area of both sides of the PCB) in the boards studied here. The higher the power dissipation, especially if high power is concentrated in a few, small components, the more important it is to have a good value of conductivity



• All the modeling was done assuming laminar flow. Because of the low power density and natural convection, the air velocities were quite low (about 0.1 m/sec). These results may not apply to cases with turbulent flow, whether the flow is forced or natural.



• Care should be taken in modeling unusual components, such as transformers, vacuum tubes, or large heat sinks. The less a component looks like a DIP or PLCC, the less likely this modeling method will work, at least for the strange component.



• Radiation has been ignored in all these cases. For real boards mounted in shelves between other hot boards, it was assumed that radiation exchange between boards and their neighbors pretty much cancels out, because they are all about the same temperature. Radiation would affect the honest components most, spreading their heat around to the rest of the board, in much the same way that conduction in the PCE does. Perhaps some of the 10 W/m/C actually includes the effect of radiation within a shelf. The resistor board study was done without heated neighbor boards, and so the resistors probably did lose some heat by radiation to the surroundings. My estimate of radiation heat loss is about 10%, which would mean that the average resistor would be about 3C hotter in the absence of radiation. (By coincidence, the model with k_PCB = 1 W/m/C overpredicts the average resistor temperature by about 3C.)



• A disadvantage of this method is that it does not give any information about component junction temperature. Because the component is modeled as a Cuboid Block with uniform power dissipation and with a fairly high conductivity, the temperature throughout the block is all the same. Because I have purposely left out detail, there is no way to distinguish between case temperature and junction temperature. This is a small dilemma for me, because what I really want to know is junction temperature, which is what determines the component reliability and functionality. What I have found is that Cuboid Block temperature corresponds to case temperature from experiment. I have to assume that the junction temperature is higher. This can be estimated in two ways: by the old fashioned q_ic method, or by constructing a much more detailed model of the particular component, and using the case temperature and other local conditions from the simple model as boundary conditions. See other papers from the 1st and 2nd FLOTHERM Users Conferences for more details.
  
   

I have presented a method for modeling arrays of Printed Circuit Boards in natural convection that predicts the case temperatures of the Important components; That method has a few simple steps:

1. separate the components into important (high power or temperature sensitive) and Trivial (low power)
2. model the components and PCB as Solve-in-Solid Cuboid Blocks
3. Important components are individual blocks
4. Trivial components are lumped together into large, uniform blocks
5. use a value of 10 W/m/C for the conductivity of the components and PCB, more or less. Less if there is less copper than "normal," more if there is plenty of copper. Everyone would like the simulation to predict the component temperatures correctly, but if there must be error in predicting, it is usually safer to overpredict than to underpredict. Conservative practice suggests using lower values of conductivity for the PCB if there is any doubt.
6. model vents, walls, grills and other parts as outlined in the FLOTHERM Users Manual, and solve the problem in the usual way

Experimental evidence (perhaps it should be called "experiential" evidence) has been presented to show some success with this method in predicting component temperature, and that the value of 10 W/m/C for PCB conductivity is a good place to start. Conductivity of components is probably not critical for accuracy, as long as it is in the neighborhood of 1 W/m/C.

My hope and my recommendation are that other FLOTHERM users try this method, and report whether it works or not. I think that it can be a handy short-cut for solving certain kinds of FLOTHERM problems, and will prove to be of some value if it is validated by the experience of other FLOTHERM users.